Horizontal speed formula. The movement of a body thrown horizontally with a speed
Consider the motion of a body thrown horizontally and moving under the action of gravity alone (neglecting air resistance). For example, imagine that a ball lying on a table is given a push, and it rolls to the edge of the table and begins to fall freely, having an initial velocity directed horizontally (Fig. 174).
Let's project the movement of the ball on the vertical axis and on the horizontal axis. The movement of the projection of the ball onto the axis is a movement without acceleration with a speed of ; the motion of the projection of the ball on the axis is a free fall with acceleration beyond the initial velocity under the action of gravity. We know the laws of both motions. The velocity component remains constant and equal to . The component grows in proportion to time: . The resulting speed is easily found using the parallelogram rule, as shown in Fig. 175. It will lean downward and its slope will increase with time.

Rice. 174. Movement of a ball rolling off a table

Rice. 175. A ball thrown horizontally with a speed has a speed at the moment
Find the trajectory of a body thrown horizontally. The coordinates of the body at the moment of time matter
To find the trajectory equation, we express from (112.1) the time through and substitute this expression in (112.2). As a result, we get
The graph of this function is shown in Fig. 176. The ordinates of the trajectory points turn out to be proportional to the squares of the abscissas. We know that such curves are called parabolas. A parabola depicted a graph of the path of uniformly accelerated motion (§ 22). Thus, a freely falling body whose initial velocity is horizontal moves along a parabola.
The path traveled in the vertical direction does not depend on the initial speed. But the path traveled in the horizontal direction is proportional to the initial speed. Therefore, with a large horizontal initial velocity, the parabola along which the body falls is more elongated in the horizontal direction. If a jet of water is fired from a horizontally located tube (Fig. 177), then individual particles of water will, like the ball, move along a parabola. The more open the tap through which water enters the tube, the greater the initial velocity of the water and the farther from the tap the jet gets to the bottom of the cuvette. By placing a screen with parabolas pre-drawn on it behind the jet, one can verify that the water jet really has the shape of a parabola.

Rice. 176. Trajectory of a body thrown horizontally
Consider the motion of a body thrown horizontally and moving under the action of gravity alone (neglecting air resistance). For example, imagine that a ball lying on a table is given a push, and it rolls to the edge of the table and begins to fall freely, having an initial velocity directed horizontally (Fig. 174).
Let's project the movement of the ball on the vertical axis and on the horizontal axis. The movement of the projection of the ball onto the axis is a movement without acceleration with a speed of ; the motion of the projection of the ball on the axis is a free fall with acceleration beyond the initial velocity under the action of gravity. We know the laws of both motions. The velocity component remains constant and equal to . The component grows in proportion to time: . The resulting speed is easily found using the parallelogram rule, as shown in Fig. 175. It will lean downward and its slope will increase with time.

Rice. 174. Movement of a ball rolling off a table

Rice. 175. A ball thrown horizontally with a speed has a speed at the moment
Find the trajectory of a body thrown horizontally. The coordinates of the body at the moment of time matter
To find the trajectory equation, we express from (112.1) the time through and substitute this expression in (112.2). As a result, we get
The graph of this function is shown in Fig. 176. The ordinates of the trajectory points turn out to be proportional to the squares of the abscissas. We know that such curves are called parabolas. A parabola depicted a graph of the path of uniformly accelerated motion (§ 22). Thus, a freely falling body whose initial velocity is horizontal moves along a parabola.
The path traveled in the vertical direction does not depend on the initial speed. But the path traveled in the horizontal direction is proportional to the initial speed. Therefore, with a large horizontal initial velocity, the parabola along which the body falls is more elongated in the horizontal direction. If a jet of water is fired from a horizontally located tube (Fig. 177), then individual particles of water will, like the ball, move along a parabola. The more open the tap through which water enters the tube, the greater the initial velocity of the water and the farther from the tap the jet gets to the bottom of the cuvette. By placing a screen with parabolas pre-drawn on it behind the jet, one can verify that the water jet really has the shape of a parabola.
 112.1. What will be the speed of a body thrown horizontally at a speed of 15 m/s after 2 seconds of flight? At what moment will the velocity be directed at an angle of 45° to the horizontal? Ignore air resistance.
112.1. What will be the speed of a body thrown horizontally at a speed of 15 m/s after 2 seconds of flight? At what moment will the velocity be directed at an angle of 45° to the horizontal? Ignore air resistance.
112.2. A ball rolled down from a table of height 1m fell at a distance of 2m from the edge of the table. What was the horizontal speed of the ball? Ignore air resistance.
Updated:
Using several examples (which I initially solved, as usual, on otvet.mail.ru), we will consider a class of problems of elementary ballistics: the flight of a body launched at an angle to the horizon with a certain initial speed, without taking into account air resistance and the curvature of the earth's surface (that is, the direction free fall acceleration vector g is assumed to be unchanged).
Task 1. The flight range of the body is equal to the height of its flight above the Earth's surface. At what angle is the body thrown? (in some sources, for some reason, the wrong answer is given - 63 degrees).
Let's denote the flight time as 2*t (then during t the body rises, and during the next interval t it descends). Let the horizontal component of the velocity be V1 and the vertical component V2. Then the flight range S = V1*2*t. Flight altitude H \u003d g * t * t / 2 \u003d V2 * t / 2. Equate
S=H
V1*2*t = V2*t/2
V2/V1 = 4
The ratio of vertical and horizontal speeds is the tangent of the required angle α, whence α = arctan(4) = 76 degrees.
Task 2. A body is thrown from the Earth's surface with a speed V0 at an angle α to the horizon. Find the radius of curvature of the body trajectory: a) at the beginning of the movement; b) at the top of the trajectory.
In both cases, the source of the curvilinear motion is gravity, that is, the free fall acceleration g, directed vertically downwards. All that is required here is to find the projection g, perpendicular to the current velocity V, and equate it to the centripetal acceleration V^2/R, where R is the desired radius of curvature.
As can be seen from the figure, to start the movement, we can write
gn = g*cos(a) = V0^2/R
whence the desired radius R = V0^2/(g*cos(a))
For the upper point of the trajectory (see figure) we have
g = (V0*cos(a))^2/R
whence R = (V0*cos(a))^2/g
Task 3. (variation on a theme) The projectile moved horizontally at a height h and exploded into two identical fragments, one of which fell to the ground in time t1 after the explosion. How long after the first piece falls will the second one fall?
Whatever vertical velocity V the first fragment acquires, the second one will acquire the same vertical velocity in absolute value, but directed in the opposite direction (this follows from the identical mass of the fragments and conservation of momentum). In addition, V is directed downward, because otherwise the second fragment will arrive on the ground BEFORE the first one.
h = V*t1+g*t1^2/2
V = (h-g*t1^2/2)/t1
The second one will fly up, lose vertical speed after the time V/g, and then after the same time will fly down to the initial height h, and the time t2 of its delay relative to the first fragment (not the flight time from the moment of explosion) will be
t2 = 2*(V/g) = 2h/(g*t1)-t1
updated on 2018-06-03
Quote:
A stone is thrown at a speed of 10 m/s at an angle of 60° to the horizontal. Determine the tangential and normal acceleration of the body after 1.0 s after the start of movement, the radius of curvature of the trajectory at this point in time, the duration and range of the flight. What angle does the total acceleration vector form with the velocity vector at t = 1.0 s
The initial horizontal speed Vg = V*cos(60°) = 10*0.5 = 5 m/s, and it does not change during the entire flight. Initial vertical velocity Vв = V*sin(60°) = 8.66 m/s. The flight time to the highest point is t1 = Vv/g = 8.66/9.8 = 0.884 sec, which means the duration of the entire flight is 2*t1 = 1.767 sec. During this time, the body will fly horizontally Vg * 2 * t1 = 8.84 m (flight range).
After 1 second, the vertical velocity will be 8.66 - 9.8*1 = -1.14 m/s (downwards). This means that the angle of velocity to the horizon will be arctan(1.14/5) = 12.8° (down). Since the total acceleration here is unique and unchanged (this is the acceleration of free fall g pointing vertically downwards), then the angle between the velocity of the body and g at this point in time will be 90-12.8 = 77.2°.
Tangential acceleration is a projection g to the direction of the velocity vector, which means it is g*sin(12.8) = 2.2 m/s2. Normal acceleration is a projection perpendicular to the velocity vector g, it is equal to g*cos(12.8) = 9.56 m/s2. And since the latter is related to the speed and radius of curvature by the expression V^2/R, we have 9.56 = (5*5 + 1.14*1.14)/R, whence the required radius R = 2.75 m.
 The body can be thrown in such a way that its initial velocity v0 will be directed horizontally (α = 0). This is the direction, for example, of the initial velocity of a body detached from a horizontally flying aircraft. It is easy to understand which trajectory the body will move along. Let us turn to Figure 15, which shows the parabolic trajectory of a body thrown at an angle α to the horizon. At the highest point of the trajectory of the parabola, the velocity of the body is precisely directed horizontally. As we already know, beyond this point the body moves along the right branch of the parabola. Obviously, any body thrown horizontally will also move along the branch of the parabola.
The body can be thrown in such a way that its initial velocity v0 will be directed horizontally (α = 0). This is the direction, for example, of the initial velocity of a body detached from a horizontally flying aircraft. It is easy to understand which trajectory the body will move along. Let us turn to Figure 15, which shows the parabolic trajectory of a body thrown at an angle α to the horizon. At the highest point of the trajectory of the parabola, the velocity of the body is precisely directed horizontally. As we already know, beyond this point the body moves along the right branch of the parabola. Obviously, any body thrown horizontally will also move along the branch of the parabola.
The trajectory of motion of bodies thrown horizontally or at an angle to the horizon can be visually studied in a simple experiment. A vessel filled with water is placed at a certain height above the table and connected with a rubber tube to a tip equipped with a tap. The emitted jets of water directly show the trajectories of the movement of water particles. Thus, it is possible to observe trajectories at different values of the angle of incidence α and velocity v0.
The time of motion of a body thrown horizontally from a certain initial height is determined only by the time necessary for the free fall of the body from this initial height. Therefore, for example, a bullet fired by a shooter from a gun in a horizontal direction will fall to the ground at the same time as a bullet dropped by chance at the time of the shot (provided that the shooter drops the bullet from the same height at which it is in the gun at the time of the shot!. .). But a dropped bullet will fall at the shooter's feet, and a bullet fired from a gun barrel will fall many hundreds of meters from him.
Problem solution example
This example was chosen for the reason that the problem under consideration is of a rather general nature and allows, using the example of its solution, to better understand all the features of the motion of a body under the action of gravity.
Initial assumptions imposed on the conditions for solving the problem
In solving this problem, we will use only two initial assumptions:
- we will neglect the dependence of the absolute value of the free fall acceleration vector on the height at which the body is at any moment of motion (see Fig. 11 and commentary to it)
- we will neglect the curvature of the earth's surface when analyzing the movement of the body (see Fig. 11 and commentary to it)

The task:
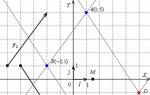
A body is thrown from a point with coordinates x 0 , y 0 at an angle α 0 to the horizon with a speed v 0 (see Figure 16). Find:- position and speed of the body after time t;
- flight path equation;
- normal and tangential accelerations and the radius of curvature of the trajectory at the moment t;
- total flight time;
- the highest lifting height;
- the angle at which the body must be thrown so that the height of its rise is equal to the flight range (provided that x 0 \u003d y 0 \u003d 0).
Solution
Let's direct the axes of the rectangular coordinate system X and Y along the directions of the horizontal and vertical displacements of the point. Since the gravitational acceleration vector does not have a component parallel to the X axis, that is, the vector equations of motion of the body have the form:
In explicit form, the expression for the projections of the vector quantities included in the first equation on the axes of the coordinate system has the form that determines the position of the body at time t:
Since each vector can be represented as the sum of its projections (these are also vectors) on the coordinate axes, each vector equation can be represented as two vector equations, but for projections. Having expressed the projections of the vector quantities included in the second equation on the axes of the coordinate system, we find the velocity components
and the expression for the resulting velocity (using the Pythagorean theorem) The tangent of the angle between the direction of the resulting velocity and the X-axis is equal, that is, it changes over time. This is understandable, since the velocity value has a geometric interpretation in the form of the tangent of the slope of the tangent to the dependence of the coordinate or radius vector on time.Eliminating t from both equations that determine the position of the body at time t, we obtain the flight path equation
To determine the tangential and normal accelerations of the body at a point with coordinates x, y, we note that the total acceleration of the body is always directed downward and represents only the acceleration of gravity, (there are no other forces and accelerations according to the condition of the problem) . The tangential acceleration is equal to the projection of the vector onto the tangent to the trajectory (i.e. −g sinγ , as seen in the explanatory figure for the problem), and the acceleration normal to the tangent is equal to the projection of −g cosγ (see Fig. 16)
thenLet's find along the way the approximate value of the radius of curvature (R) of the trajectory at the moment t. Assuming that the point moves along an arc of a circle (this is an approximation that simplifies the final mathematical formula of the result, which actually does not take place and is best performed near the point of maximum body lift), we use the formula
thenIf the body is thrown from a point on the surface where and y = 0 , the problem becomes much simpler. Reducing by (x max − x 0) , we find that
The total flight time can be determined from the formula ![]() where
where
The greatest lifting height of the body is reached at the moment t when v y = 0 . Since the component of the velocity vector along the Y axis is , then at the point of maximum rise of the body, the equality v y = 0 takes place, from which we obtain